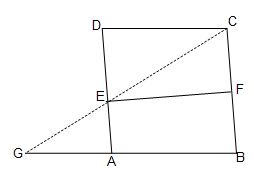
E and F are respectively the mid-points of the non-parallel sides AD and BC of a trapezium ABCD.Prove that EF is parallel to AB and EF=1/2(AB+CD)
CONST. - Extend DC to point P where it meets a line drwn from point B through point E .
PROOF - In triangles DEP and AEB ,
AngleDEP = AngleAEB (vert.opp.angles)
AE=ED(E is the mid point of AD)
AngleBAE=AngleEDP(alt. angles)
Therefore, by ASA congruence , triangle ABE is congruent to triangle PED .
Hence AB=DP BE=EP(C.P.C.T.)
Therefore, E is the mid point of BP .
Now, in triangle BPC ,
E F are the mid points of PB BC respectively .
Therefore EF is parallel to CD EF = 1/2 CP
EF = 1/2 CP
EF=1/2 (CD + DP )
EF=1/2 (CD + AB) as AB =DP.
Hence , proved .